Snow zones map - Belgium

The Belgian territory is not divided (so there is no map in the national annex). sk₀ = 0.50 kN/m²

Snow loads are calculated as follows:

Up to 100m

From 100m to 700m

where A is the elevation of the site.

P_n is the annual probability of exceedence :

dwl is the design working life, in years.

V is the coefficient of variation of annual maximum snow load, calculated as follow :

It should not be applied for annual probabilities of exceedence greater than 0,2 (i.e. return period less than approximately 5 years).

See the features of Eurocodes Zoning for snow analysis in Belgium

Available in English/French, otherwise «Google Translate»!

Coordinates in World Geodetic System 1984 (WGS84) 4.9053°, 50.2445°

Coordinates in Belgian Geodetic System 2008 (Lambert 2008) 688279m, 603864m

Address Rondchêne, 5500 Dinant, Wallonie

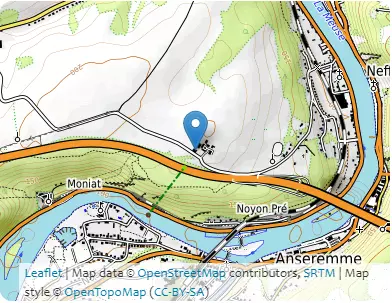
Coordinates	4.9013°, 50.241°	4.9044°, 50.2436°
Elevations	96m	189m
Obstacle effective height H	93m
Slope actual length L_u / L_d	361.4m
Slope angle Φ	25.7%
Horizontal distance site/top x	121.2m
Elevation at the place of construction	197m

Type of building Design working life category Max height Orientation from North

common building with floors (apartments and/or office spaces)

100years

7.0m

35°

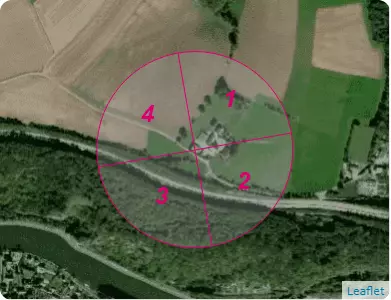
Sectors	1	2	3	4
Categories	II	II	III	II

Radius R of the angular sector 300m

NBN EN 1991-1-3 ANB (october 2007)

Zone Belgium : (s_k,0 = 0.5 kN/m²)

Criteria for zoning : Dinant, Namur

Characteristic value of snow on the ground at the relevant site : s_k,197 = 0.613 kN/m²

Ground snow load with a return period of 100 years : s_{100 ans} = 0.695 kN/m²

NBN EN 1991-1-4 ANB (december 2010)

Zone : 24.0 m/s

Criteria for zoning : Dinant, Namur

Zone c_dir : Belgium

Sectors	1	2	3	4
Sector definition	from 350 ° to 80 °	from 80 ° to 170 °	from 170 ° to 260 °	from 260 ° to 350 °
Fundamental value of the basic wind velocity v_b,0	24.0m/s
Shape parameter K	0.2
Exponent n	0.5
Annual probability of exceedence p	0.01
Probability factor c_prob	1.038
Directional factor c_dir	1.0	0.983	1.0	1.0
Basic wind velocity v_b	24.9m/s	24.5m/s	24.9m/s	24.9m/s
Reference roughness length z_0,II	0.05m
Roughness length z₀	0.05m	0.05m	0.3m	0.05m
Terrain factor k_r	0.19	0.19	0.215	0.19
Height above ground z	7.0m
Minimum height z_min	2.0m	2.0m	5.0m	2.0m
Roughness factor c_r(z)	0.939	0.939	0.678	0.939
Obstacle type	cliffs
Exposure type	-	-	downwind	-
Factor depending on the type and dimensions of the obstacle s _max	0.0	0.0	0.598	0.0
Orography factor ^* c_o(z)	1.0	1.0	1.308	1.0
Mean wind velocity v_m(z)	23.4m/s	23.0m/s	22.1m/s	23.4m/s
Turbulence factor k_l	0.995	0.995	1.247	0.995
Standard deviation of the turbulence σ_v	4.713m/s	4.634m/s	6.696m/s	4.713m/s
Turbulence intensity I_v(z)	0.201	0.201	0.303	0.201
Air density ρ	1.225kg/m³
Exposure factor c_e(z)	2.124	2.124	2.456	1.124
Peak velocity pressure q_p(z)	824.7N/m²	797.5N/m²	953.3N/m²	824.7N/m²
Peak wind velocity for Serviceability Limit States v_p(z),SLS	130.8km/h	128.6km/h	140.6km/h	130.8km/h
Peak wind velocity for Ultimate Limit States v_p(z),ULS	160.2km/h	157.5km/h	172.2km/h	160.2km/h

* The orography factor is calculated for a well individualized obstacle (an emergent zone compared to a general ground without marked relief)

NBN EN 1998-1 ANB (october 2011)

Zone : 1 (0,39 m/s²)

Criteria for zoning : Dinant, Namur

A seismic analysis may be required for this building.